KAUST�s Global Boost for Applied and Computational Mathematics
September 21, 2009
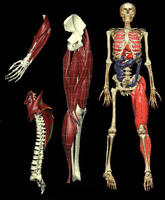
Figure 1. Components in the Physiome Project.
John Ockendon
It will not have escaped readers of SIAM News that the King Abdullah University of Science and Technology provides unprecedented support for research in applied and computational mathematics, especially through two (of a total of four) centres established by KAUST's Global Research Partnership---one at Oxford University and the other at Texas A&M University. The recent launch meeting of the Oxford Centre for Collaborative Applied Mathematics (OCCAM) offered a singular opportunity to proclaim the value of the mathematical sciences in the 21st century.
Because the meeting was organised primarily to publicise the impact that applied and computational mathematics can have outside the mathematical community, several of the senior speakers were not professional mathematicians. Their visionary presentations were backed up by shorter talks from OCCAM researchers, the whole programme being around the KAUST themes of biosciences and bioengineering; materials science and engineering; resources, energy, and the environment; and, of course, applied mathematics and computational science.
The audience, which was definitely not of the "rent-an-audience" variety, was greatly receptive to expositions in the following areas.
Physiology and Neuroscience. Peter Hunter (Auckland Bioengineering Institute) described the evolution and current status of perhaps the most practically important multiscale modelling challenges of them all---the Physiome Project (www.ngi-nz.co.nz/applications/physiome.html). Indeed, could any application of mathematics have as much impact as whole-body modelling---with its synthesis of components down to and even beyond the level of the cell? And how many clever new mathematical ideas will be needed to integrate the models for the different physiological components (some of which are shown in Figure 1) in an efficient, bottleneck-free computational framework?
At the moment, the physiome does not incorporate much input from neuroscience, largely because the mathematical modelling of the brain is so challenging. From this daunting complexity, however, Paul Bressloff (previously of the University of Utah, now at OCCAM) has been able to extract simplified dynamical models covering spatial scales from synapses to cortical networks, and even making use of ideas about symmetry in hallucinations. Optimistic as he is, Bresloff expressed no great confidence that cognition is now ripe for modelling.
These two lectures were just the first two illustrations of the role mathematics can play in addressing complex multiscale systems.
Materials Science and Engineering. L. Mahadevan (Harvard) and Dick James (University of Minnesota) provided equally stimulating food for thought in the area of multiscale materials modelling from the submicron level to the laboratory scale. Mahadevan described disordered networks that can undergo a phase transition from being a fragile network to a stiff solid when a topological order parameter crosses a dimension-dependent theoretical threshold. Such networks could be useful for describing the mechanical properties of materials, whether stiff or soft, living or non-living, that fall outside the traditional classifications of theoretical mechanics.
James has been able to identify a remarkable unification of fluid and solid mechanics for what fluids experts call "viscometric" flows. This opens new possibilities for researchers in both communities, and James was able to use carbon nanotube deformation as an illustration.
These two sessions are also relevant to OCCAM's liquid crystal research programme, which is led by Apala Majumdar. The information that LCDs give us second by second is, in many senses, key to our survival, and applied and computational maths is the key to controlling the molecular orientations on which LCDs rely.
Energy and the Environment. The two "Big E's" presented the launch meeting with its broadest range of theoretical challenges. Don Turcotte (geology, UC Davis), a renowned, yet mathematically sympathetic earth scientist, reminded us of the effectiveness of fractal distributions in collapsing multiscale data from across the whole spectrum of geophysics and even to the extent of capturing the statistics of record-breaking events. However, the discussion also revealed the somewhat alarming gulf that can exist between mathematical statistics and geostatistics.
David Mackay (physics, Cambridge) enthralled the audience with a crisp and entertaining account of the qualitative implications of different ways in which civilization might respond to the energy challenge. The implication was that the mathematical sciences have a key role to play in an enormous number of individual research projects, but Mackay's principal message was that the emphasis should be put on solar energy harvested in desert environments.*
Applied and Computational Mathematics. In the face of the real-world opportunities and challenges described at the meeting, our community clearly has to scour every nook and cranny of its tool-kit. Peter Markowich (Cambridge, and a KAUST GRP Investigator) highlighted what may be our most powerful methodology. He showed how partial differential equations can unify phenomena over almost all terrestrial scales, from Bose�Einstein condensates to tsunamis.
Although his talk revealed how much we know at distinct scales, mathematicians currently have far less to say about how to glue the different scales together. It was left to Gil Strang (MIT) to remind everyone of scary challenges like the behaviour of the spectrum of the 2-D Laplacian in a regular n-gon as n tends to infinity.
All the talks are available on the OCCAM Web site (www.maths.ox.ac.uk/groups/occam).
***
Along with these focused sessions, the launch meeting reflected OCCAM's industrial roots by including a session on mathematics in industry. Session leaders Colin Please (University of Southampton) and Graeme Wake (Massey University, Auckland) both extolled the joys of working on industrial problems---describing both the difficulties inherent in making contact with industrialists and some of the applications to which maths has really made a difference. Success stories, they said, are crucial to the promotion of maths-in-industry.
Chris Farmer (previously of Schlumberger, now at OCCAM) described key challenges for mathematicians in the oil industry, such as optimal well-placement under uncertainty and the geometric modelling of rock formations.
Further talks and discussion focussed on mechanisms for promoting the use of mathematics in solving industrial problems. Study Groups were a recurring motif; other ideas included internships in industry for postgraduate students and technology translators to help bridge the communication gap between academia and practical scientists in industry. Robert Leese (UK Knowledge Transfer Network in Industrial Mathematics) explained how his organisation has worked to facilitate the flow of information and people in all directions. Hilary Ockendon (Oxford) described the global scale of this activity, as summarised in the recently issued OECD report (www.oecd.org/dataoecd/31/19/42617645.pdf).
Complementing the scientific sessions were debates on how the centres at Oxford and Texas should interface with KAUST and on participants' visions for applied and computational maths at KAUST. These debates were led by Jim Calvin, director of OCCAM's sister centre at Texas A&M, Ahmed Ghoniem (chemical engineering, MIT), John Wettlaufer (geology and geophysics, Yale; the cold ground in Figure 2 is the target of some of his research), Julian Hunt (University College London, and our esteemed representative in the House of Lords), and, most vociferously, by David Keyes (chair of KAUST's Mathematical and Computer Sciences and Engineering Division). Aside from pedagogical issues, several themes emerged from the discussions:
- The research centres will need to focus on such pressing issues as preservation of the Red Sea coral reefs and the mathematical and computational underpinnings of climate change models.
- Agreement on the need for problem-driven workshops, such as Study Groups, gave momentum to the idea of the first Saudi Arabian Study Group with Industry, to be held in the Kingdom in 2010, with emphasis on the oil industry.
- By operating as the nerve centre of a global network of academic and industrial researchers, OCCAM is in a fantastic position to conduct collaborative, interdisciplinary research, working in tandem with computational scientists at KAUST and the new university's state-of-the art IBM petascale supercomputer.
- The most important research concern is scalability, i.e., the need for a systematic unified theory of multiscale computation in areas ranging from genomics, nanotechnology, and combustion right through to climate modelling.

Figure 2. Cold ground.
More controversial, and perhaps beyond the scope of KAUST, is the role of applied and computational mathematics in social science, especially in policy and decision making, this being a topic that will surely increase in importance. Similar uncertainty arises for neuroscience, as in the discussions of the comparative roles of large-scale computation, visualisation, and cognition. It is clear that the evolving relationship between mathematics and computer science and visualisation warrants close attention, and there is every hope that KAUST will play a leading role in developing this interface.
John Ockendon, FRS, is a professor at OCCAM and a Fellow of St Catherine's College.
*Soon afterward, your correspondent was present at an energy event at the House of Lords. The contrast in clarity between Mackay's approach and those of non-mathematically-minded government advisers could hardly be improved on as an advertisement of the need for quantitative science to have a vital role in meeting the energy challenge.

