Mathematical Models and Computational Methods for Nano- and Bio-technologies
October 21, 2007
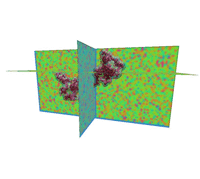
Figure 1. Single-file translocation, with accompanying velocity field and intrinsic fluctuations. Images courtesy of S. Melchionna.
Roderick Melnik
Hand in hand with the development of new nano- and bio-technologies has come an increasing need for new mathematical models that describe systems, phenomena, and processes associated with these technologies. In many cases, such mathematical models are being developed as coupled systems of differential/difference and/or integral equations that require efficient computational techniques for their solution. Scientists and technologists working in industry are often unaware of state-of-the-art developments in mathematical modeling that can be directly pertinent to their problems. At the same time, mathematicians are often unable to tackle challenging new applied problems because they lack knowledge of the domain in which the problems arise.
Through a number of representative examples, speakers in a two-part minisymposium at ICIAM '07--Mathematical Models and Computational Methods for Nano- and Bio-technologies--demonstrated how mathematical modeling tools and computational techniques can make a difference in cutting-edge developments in these technologies. Simone Melchionna of the University of Rome La Sapienza opened the session with a discussion of a methodology for modeling nano-scale systems, focusing on polymers embedded in fluctuating hydrodynamic solvents. Other members of this research group were Maria Fyta (Harvard University), Sauro Succi (IAC M. Picone, National Research Council), and Efthimios Kaxiras (Harvard).
The researchers conducted a thorough numerical study of biopolymers translocating across nanopores, mimicking a device for automatic DNA sequencing. Examples are shown in Figure 1, which illustrates a single-file translocation with accompanying velocity field and intrinsic fluctuations, and Figure 2, which illustrates a double-file translocation with accompanying fluid�
atom synergy. The translocation time was found to show a power law dependence on the polymer length, with an exponent in agreement with experimental measurements. The group's simulations accounted for the coupling of the correlated molecular motion to hydrodynamics and revealed scaling behavior far richer than that observed with a mean-field model.
Jack Tuszynski of the Cross Cancer Institute in Edmonton, Canada (co-organizer of the session) discussed the role of nonlinearities in mathematical models describing the properties of several key biomolecules--actin filaments, microtubules, and ions surrounding them. The model he presented, based on a system of coupled differential equations, describes the interactions between ions in the solution and the filaments they surround. Myosins, important motor proteins that move along actin filaments, surfaced in the talk of Hannes Bolterauer of the University of Giessen, Germany, who discussed several interesting models describing their dynamics. He also presented several models for kinesin, a nanometer-scale motor protein that "walks" along the surface of microtubules. Both of these speakers emphasized the importance of coupled dynamic models in studying the properties of motor proteins with applications to bio-technological innovations.
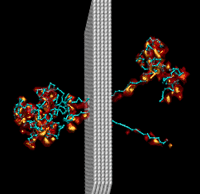
Figure 2. Double-file translocation with accompanying fluid�atom synergy.
Robert Anderssen presented joint work with his colleague Frank de Hoog of the Commonwealth Scientific and Industrial Research Organization in Canberra on the interconversion integral equation for relaxation and creep. In the talk Anderssen focused on a mathematical model for determining rheological phenotypes, which are playing an increasingly important role in biotechnology. He emphasized the importance in this study of taking into account the viscoelastic nature of biomaterials and presented a number of results from recent mathematical investigations.
Discussing coupled nonlinear effects in nanostructures and their applications, Roderick Melnik (co-organizer of the minisymposium) presented joint work with Waterloo colleagues Roy Mahapatra, Niraj Sinha, and John Yeow, with David Jaffray (Princess Margaret Hospital), Morten Willatzen and Benny Lassen (both of the University of Southern Denmark), and Lok Lew Yan Voon (Wright State University, USA). In a number of the examples presented, mathematical models must take such effects into account for an adequate prediction of nanostructures' properties; the main structures considered were low-dimensional semiconductor nanostructures and carbon nanotube-based devices.
Riccardo Sacco (Politechnico di Milano, Italy) discussed computational models for the numerical simulation of voltage-operated ionic channels in nano-bio-electronics, joint work with Bice Chini (National Research Council, Italy), Joseph Jerome (Northwestern University, USA), and two colleagues from Milano, Massimo Longaretti and Giovambattista Marino. Sacco presented a novel mathematical model of such channels, motivated by their applications in nano-bio-electronics. The core of the model is based on a coupled system of partial differential equations: a Poisson�Nernst�Planck system that allowed the researchers to account for electro-chemical phenomena and a Navier�Stokes system that allowed them to account for fluid-mechanical phenomena. Sacco presented numerical results for realistic voltage-operated ionic channels.
In summary, the minisymposium demonstrated some of the key mathematical and computational challenges associated with state-of-the-art developments in nano- and bio-technologies, with the emphasis on important representative examples. At the same time, it exposed the more technologically oriented part of the community to the most advanced mathematical and computational techniques currently being developed, encouraging interdisciplinary collaborations. Indeed, the development and integration of mathematical modeling techniques into nano- and bio-technologies is an essential prerequisite for further progress in this increasingly interdisciplinary field.
This minisymposium at ICIAM '07 was the third in a series of meetings initiated in 2005 and devoted to the mathematical modeling of coupled processes and phenomena for new technologies. The next workshop in this series will be held in 2008 in Venice, Italy, as part of the WCCM/ECCOMAS meeting.
Roderick Melnik is a professor and Tier I Canada Research Chair in the Department of Mathematics at Wilfrid Laurier University, Waterloo, Canada.

