Prize-winning Video Brings M�bius Transformations to Life
December 17, 2007
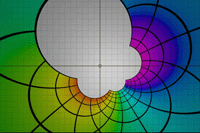
The image of the unit square under a M�bius transformation. The transformation maps the square conformally onto an exterior region whose boundary consists of four circular arcs, the images of the edges of the square.
Michelle Sipics
Each year since 2003, Science magazine and the National Science Foundation have sponsored a contest, challenging researchers to find new ways of visualizing scientific data. As their motivation, the sponsors cite the role that "bringing data to life visually" can play in promoting both better understanding of scientific concepts in the public and communication between scientists working in different disciplines.
As in previous years, math was in the spotlight in this year's competition, thanks in part to the contribution of mathematicians Douglas Arnold and Jonathan Rogness of the University of Minnesota. Their entry, "M�bius Transformations Revealed," tied for an honorable mention in the competition's noninteractive media category. The short video, set to Schumann's Kinderscenen, shows animated M�bius transformations seen from both a two- and a three-dimensional viewpoint.
(In 2006, awards to mathematicians and their entries ranged from honorable mentions to first in their respective categories. See "The Human Heart Laid Bare" and "Visualizing Mathematical Surfaces," SIAM News, November 2006; http://www.siam.org/news/.)
Arnold and Rogness's video should prove useful to mathematics students everywhere. As Arnold points out, "M�bius transformations are the simplest non-polynomial maps of the complex plane, and an excellent source of conformal maps. . . . They are studied in virtually any class on complex analysis." But what encouraged Arnold and Rogness to create the video in the first place?
Appropriately, it was a complex journey.
"I had produced a much simpler animation of a M�bius transformation for a class I gave back in 1997," explains Arnold, who has been director of the Institute for Mathematics and its Applications since 2001. A Canadian film director named Jean Bergeron, who was making a documentary on the mathematics in the work of the Dutch artist M.C. Escher, found Arnold's ten-year-old animation on the Web.
This January, Bergeron asked if he could use those old animations, which would have to be reproduced at higher resolution, Arnold recalls. "I did that, but it got the idea of depicting M�bius transformations through animation back in my mind."
Enter Jonathan Rogness, who has expertise in developing mathematical applets for educational use. An assistant professor of mathematics, he also works in the university's Institute of Technology Center for Educational Programs.
"I like to use applets when I think it can help students understand or visualize a tricky concept," Rogness says. "When using vector fields to talk about fluid flow, for example, we'll use an applet which lets us watch the particles move around, analyze their trajectories, and so on."
Always on the lookout for nice visualization projects, Rogness says that Arnold's idea appealed to him for two reasons.
First, "M�bius transformations result in some very striking images, and I thought it could be a way to convey the beauty of mathematics to non-mathematicians," he explains. "Second, I felt this could help students get a better understanding of M�bius transformations. Textbooks might show a picture of a square turned inside-out by a M�bius transformation, but it can be hard for students to understand how the lines in the original square morphed into the resulting picture.
"Sometimes as mathematicians we forget that things which seem clear or obvious to us are not at all clear to non-professionals," Rogness adds.
With the animations of M�bius transformations, the video depicts a theorem that Arnold says he learned only recently: "All M�bius transformations can be obtained through inverse stereographic projection, followed by a rigid motion of the sphere of projection, followed by forward stereographic projection back to the plane." If you had to read that through a few times, you'll appreciate the video. A single viewing suffices to explain why the judges of the 2007 Science and Engineering Visualization Challenge chose to honor it: Just a few minutes of video beautifully illustrate the 29-word theorem.
The video's reach extends beyond Science subscribers: It has also been viewed more than 60,000 times on YouTube as this issue of SIAM News goes to press. What's more, a portion of "M�bius Transformations Revealed" appears in Bergeron's recently released film.
At the end of May, Arnold sent the just completed animation for "M�bius Transformations Revealed" to Bergeron. Bergeron replied, "Wow! Very interesting indeed . . . [but] I am in my last days of final edit." An hour later, he wrote again: "Your rendering is truly beautiful, as compared to any previous versions. Ah! I wonder if I could take the time to re-visit the final edit."
At that point, Bergeron decided that he would use a portion of "M�bius Transformations Revealed" in his film, rather than the re-rendered animations from 1997---but only if Arnold and Rogness could re-render their video in HD within 24 hours.
"That meant producing about 500 frames, for a total of about 3GB of data," Arnold says. He and Rogness offered their terms.

When viewed in three dimensions, the image of the square under the M�bius transformation can be obtained by stereographic projection from a sphere that has been rotated and translated after the square has been projected onto it. Video frames courtesy of Doug Arnold and Jonathan Rogness.
"Yes, we would do it, but our payment was that he would hold the U.S. premiere at the IMA. He agreed, and his film, titled Achieving the Unachievable, was shown for the first time in the U.S. on November 1, at the IMA, with Bergeron present for a Q&A at the end."
That's how some ten-year-old animations for a mathematics course turned into an award-winning video, which then made its way into a documentary on the solution of a math problem involving the work of M.C. Escher.
Michelle Sipics is a contributing editor at SIAM News.

