In the Mix at Model REU: Creative Mentor, Talented Students, Hand-Matched Problems
July 25, 2004

Each summer for close to three decades, Joe Gallian (left) has run a widely emulated undergraduate research program at the University of Minnesota Duluth. Shown here with him are 2000 participants (from left) Sarah Moss, Manish Patnaik, David Moulton (a 1987 participant and now a yearly visitor), Mike Develin, Ben Reichardt, and Thomas Carlson.
Sara Robinson
In the world of professional sports, the most talented athletes aren't always the best coaches. The same is true of mathematicians, says Joe Gallian, a professor of mathematics at the University of Minnesota Duluth, who has devoted his professional career to coaching mathematically talented undergraduates.
"I'm not a whiz kid, I'm not an incredibly strong mathematician," Gallian says. "But my talents match up with what strong students don't have. I contribute more to the math community as a coach than through my research."
Gallian directs a mathematical "summer camp" on the Duluth campus, one of the several dozen math REU (Research Experiences for Undergraduates) programs funded by the National Science Foundation at colleges and universities around the U.S. In operation since the late 1970s, the Duluth program is the oldest and perhaps best known of the math REUs; it's also notorious for being the toughest to get into, accepting only six to nine of the 80 to 90 students who apply each year. Twenty-two Duluth participants had been Putnam fellows, and 21 had been members of International Mathematical Olympiad teams.
To each of these talented students, Gallian assigns a problem, hard enough that the results will be publishable yet accessible enough to be understood by an undergraduate. Over the 10 weeks of the program, he gives the students the encouragement they need to keep plugging away, even though they may not be making much progress. By the end of the summer, most of the students have full or partial results on their problems and he sets them to work writing up the results for publication. A typical student leaves Duluth with a singly authored research paper that will eventually appear in a well-regarded research journal. Many of the students give talks on their work at the Joint Mathematics Meetings the following winter.
"There are outstanding students who can do professional-level work, but they don't know how to get started," Gallian says. "They don't know what problems are open, and they need pep talks. Even outstanding students tend to think they can't do original research."
About 80 percent of Gallian's REU students go on to complete doctoral degrees in mathematics, and a significant proportion of the early alumni now hold research positions in university math departments or government think tanks. Most who don't continue in math get PhDs in related fields, such as economics, physics, or computer science, Gallian says, although a few have bypassed advanced degrees for careers at technology companies or on Wall Street. Two Duluth participants have received Clay Mathematics Institute Long-term Prize Fellowships, and two have been awarded five-year fellowships from the American Institute of Mathematics.
What is the recipe for Gallian's success? By most accounts, the key ingredient of the program is Gallian himself: "He offers advice as you need it, but at the same time he's really good at stepping back and sort of letting you figure things out," says Aaron Abrams, who participated in the program in 1992. "It's really up to you to make it what you want."
Participants describe Gallian as having high expectations, yet as helpful and supportive. A warm and lively mentor, he loves to tell stories and does everything he can to make sure the students have fun as well as produce first-rate research.
"He has this enthusiasm that's very contagious and people get very excited about what they're working on," says Manjul Bhargava, who participated in the program in 1995, received a PhD from Princeton University in 2001, and is now a full professor at Princeton. "It was one of the most enjoyable and productive summers I've ever had."
Creating Colleagues
In 1972, when Gallian arrived in Duluth, students had to knock on doors to find faculty supervisors for their senior projects. Gallian welcomed the opportunity to advise students and soon found himself with disproportionate numbers of them. Several years later, he read an article in the Notices of the AMS announcing NSF funding for undergraduate research programs (URPs) and decided to apply.
"I thought it would be fun to work with outstanding students from across the country," Gallian says. "Duluth is isolated; no one else there did research in math. I didn't have any colleagues to work with, and I thought it would be an opportunity to have them."
The Duluth program ran for the first time in 1977, with six students. In the early years, Gallian would give the students problems related to his own research. "That's the way I started," he says, "but eventually they were eating up the problems faster than I could think about them myself."
In the early 1980s, the URP program was eliminated, but the Duluth program survived with local funding. In 1985, when NSF resurrected the program, renamed REU, Gallian received a grant, as he has ever since. Every three years, Duluth must compete afresh with dozens of other proposals, about a third of which are funded, says Lloyd Douglas, who allocates REU funding for NSF's Division of Mathematical Sciences. Duluth is continually funded because of its excellent track record, Douglas says.
NSF is not Gallian's only source of funding. Duluth is also one of several undergraduate research programs funded by the National Security Agency, which also has its own highly competitive summer research program---the Director's Summer Program---modeled on Gallian's. Gallian often recommends that Duluth REU graduates who have received PhDs consider careers at NSA or the Center for Communications Research, which is part of the Institute for Defense Analyses, a federally funded intelligence think tank. If the students express interest, he writes them letters of recommendation; every student Gallian has recommended has been offered a position. Other former Duluth participants now working in academia, including Bhargava, have visited CCR for summer stints.
Duluth alumni also visit Gallian's program, many of them year after year. These former students have become not only the colleagues he was seeking, but also his good friends. He has published papers with them, and he was the best man at one former participant's wedding. The students, in turn, have become part of a network whose members help one another professionally, and who help Gallian by suggesting problems or recommending students. Fifteen or so former Duluthians typically convene at the Joint Mathematics Meetings each year for a dinner.
"Now I know a lot of young mathematicians all over the country and I know them well," says David Moulton, a 1987 participant who has retained strong ties to the program and to Gallian.
"Joe has created a gigantic family," Abrams adds.
The Duluth Experience
Over the years, the Duluth REU has evolved from an activity run by Gallian for a few students into a large program with multiple instructors and an annual budget of approximately $100,000. This year, nine students are working on problems with the assistance of a stream of visitors-former students who spend a week or two in Duluth-and two research advisers, also former participants, who stay for the entire ten weeks.
The advisers and visitors share three-bedroom suites on the campus with the students. The suites are adjacent, which gives them the feel of one large apartment.
Aaron Abrams recalls being shown to his room in 1992 and finding on his desk a letter to Gallian from Peter Johnson, a professor at Auburn University. The letter suggested a problem related to an attached journal paper. "That first night I was there I read the paper and tried to understand it," Abrams says. "My roommates in their rooms were doing the same."
Once the program is in full swing, student life centers around the apartments. The students spend a lot of time on their own, trying to make progress on their problems. Some work in their rooms, and others go to the library, the lab, or some other spot they find conducive to thinking. Bhargava recalls finding inspiration under a favorite tree next to a stream in the woods.
Though the students talk to one another about their problems and get feedback and suggestions from the advisers and visitors, they do not work in groups. "I want them to have ownership," Gallian says. "I stress cooperation, not competition."
For breaks, students gather in the common areas for cards or board games, or go out for lunch or a walk or run by the lake. In the evenings, they cook together in the suite kitchens. On weekends, in the vans Gallian rents for their use, they can grab pizza and a movie or go bowling or miniature golfing.
Although most of the time is unstructured, the students join Gallian for lunch on Mondays and Tuesdays, gathering afterward in a classroom for short talks on each student's progress. Wednesdays are devoted to mandatory all-day field trips, typically in one of the beautiful parks surrounding Duluth. Each year's activities include rafting, kayaking, hiking, biking, climbing, and alpine sliding. Watching the sun rise over Lake Superior is a program tradition.
The visitors and advisers participate in all the activities, and spend time talking with the students about their problems. Gallian no longer works directly with the students on their research: "The visitors are smarter than I am and, collectively, they have a lot of knowledge," he says. "Even if eight of the visitors know nothing about a particular subject, the ninth or tenth one does."
David Moulton, who is now at CCR in Princeton, visits the Duluth program every year. As with the other visitors, Gallian's grant funds only Moulton's travel expenses. CCR gives him a per diem and the necessary time off.
Moulton seeks out each student in turn to get an idea of what they're working on, acting as a sounding board for their ideas and offering suggestions when he can. Working with the students on their problems is both challenging and stimulating to his own research, he says.
"I always look forward to going back," Moulton says. "It's fun to see Joe and other old friends, and Joe always gets students who are interesting and have good personalities."
Melanie Wood, a 2000 participant, returned as a visitor for the next three summers and is one of this summer's two advisers. Advisers and visitors play similar roles, she says, except that the advisers must make sure each student gets attention; they also give Gallian logistical support and provide him with progress reports on each student.
With so many skilled helpers, Gallian can focus on one of his primary roles: that of cheerleader. Even the best students sometimes get stuck and lose confidence; Gallian takes these students aside and draws on his store of anecdotes about students in similar situations who managed to turn things around. "'Keep hustling and something good will happen'---that's one of my famous phrases," he says. He also uses the pending arrival of visitors as a source of encouragement, promising that a student will be the first to talk to a particular person.
About half the time, a student's initial problem doesn't work out, Gallian says. The problem might not capture the student's interest, or it might be too hard or too easy. In such cases, Gallian will give the student a different problem.
Abrams recalls that he loved his initial problem, which had to do with a type of chromatic number for subsets of Euclidean space, although a solution proved elusive. After weeks of trying different techniques and talking to visitors, he hadn't made any progress. Finally, having tried everything he could think of, Abrams asked Gallian for a different problem. But he never found the new one as appealing as the original, to which he kept returning in his mind.
In the middle of the summer, he and two other students got permission to drive to Massachusetts to attend a reunion for the high school math camp at Hampshire. There, he met a former participant in the Duluth program, who, on hearing about the first problem, offered an idea that seemed promising. When Abrams got back to Duluth, he set about trying to make it work. "Using that idea and a couple of others I'd had, I actually solved the problem three days before the program ended," he says. "It all happened in a flurry at the end after weeks of no progress." He saved the last-minute success as a surprise for his final talk and later published his work in the journal Discrete Mathematics.
Like several other former Duluth students, Abrams, who will start a tenure-track position in the math department at Emory University this fall, is supervising a research program for undergraduates. His program, at the University of Georgia in Athens, is in many ways like Gallian's.
Matching Students to Problems
Former participants in the Duluth program credit Gallian for his "uncanny ability" to find problems that are not only interesting and accessible but also appropriate for individual students. "A lot of people could cheerlead the ways he does," says Moulton. "I think the most distinguishing talent he brings to the program is his choice of problems and the matching of them with students."
Though Gallian delegates many aspects of the program to his disciples, problem selection is one task he does not relinquish. To find problems, he looks at math archives, goes to talks and conferences, solicits suggestions from former participants and colleagues, and reads journals. He sticks to graph theory and combinatorics---topics that tend to be accessible to undergraduates. Occasionally, he finds a good problem in algebra. Within these areas, he looks for problems whose solution is likely to require cleverness, but little background.
By March in a given year, he has collected 60-80 problems to sort through and grade for appropriateness. Eventually, he narrows the list to a dozen or so.
He assigns the hardest problems first, leaving the easier problems as backups for students who initially don't make progress. It's rare that a student spends the whole summer on one problem: Some students finish in three or four weeks, and in a typical group several students get stuck, requiring new problems at least once. Nearly every summer, Gallian says, there is one student who is not successful at research, even with the easier problems.
Gallian has the students write up their results in a form appropriate for journal submission, and he identifies journals that would be appropriate for particular papers. He prefers that the students complete manuscripts before they leave Duluth, giving the advisers and visitors time to read them over. Typically, though, the students don't finish and Gallian works with them remotely for another year or even two.
"This is another place where being a cheerleader is important," Gallian says. "Even very good papers get rejected-a lot of serendipity is involved. Then I have to tell them that their paper is worthy of publication." More than a hundred papers by Duluth students have been accepted for publication, most of them in major journals (a program bibliography can be found at http://www.d.umn.edu/~jgallian/).
Finding a Good Mix
Creating an environment that is conducive to research requires attracting and choosing a good mix of students. The students have to be very strong mathematically, capable of working independently on hard problems, but their personalities are just as important, Gallian says. He looks for students who are lively and fun-loving and who will interact harmoniously in close quarters.
The program's long history and strong reputation ensure an annual pool of mostly strong applicants. Immediately after the February deadline, Gallian pares the initial 80 some applicants down to 20. This group is so strong that their course grades don't tell him much. Instead, he looks for students from strong undergraduate programs who have done something extra-completed an advanced college-level math course while in high school, say, or performed well on the Putnam, participated in the Mathematical Olympiad training program, or won an Intel (formerly Westinghouse) scholarship. He also favors students who have participated in the Hampshire or PROMYS (at Boston University) programs for high school students, or who have spent a semester in the Budapest combinatorics program. For the final cut, Gallian aims for diversity: He likes the group to include women and a few students who are not from major research universities.
Although his NSA and NSF funding can be used only for American citizens, Gallian typically accepts one strong international student; he has funds he can use for their travel and living expenses, and those students often obtain stipends from their undergraduate institutions.
In addition to recommendation letters, Gallian relies on his network of 118 for-mer program participants to give him feedback about applicants' personal qualities. He says he has turned down Putnam fellows who have the reputation of being difficult and who he feared would not fit well socially with others. "My program is small enough that I have to choose people who it would be fun to spend the summer with," Gallian says.
Moulton, who was a student at Berkeley, had participated in the Olympiad training program and in the Budapest program. Bhargava, from Harvard, had won a New York State high school science competition, had finished in the top 20 on the Putnam, and had been rated the best of the 20 students in the NSA Director's Summer Program the previous summer. Wood, from Duke, had been the first woman to represent the U.S. in the International Olympiad (and after participating in the Duluth program was the first American woman to be a Putnam fellow). Abrams, who did his undergraduate work at the University of California, Davis, had participated in the Hampshire program and had taken the Putnam, though he says he didn't perform particularly well. He says he thinks he was accepted because he was already friends with some of the other participants and Gallian thought they would be a good group.
Despite the careful screening process, Gallian says that his batting average is not perfect. The program does not work for some students, he says, either for personal reasons or because he could not find the right problem for them.
The Results
A critic might ask whether there is a need to train the mathematically talented. Won't Putnam fellows and Olympiad champions ease into productive research careers without help?
Perhaps, says Moulton, who has heard such criticisms, but that doesn't mean the program isn't useful. Some students are good at mathematics but don't know if they want to do it for a living, he adds. Moreover, the program gives the superstars early exposure: "There are some people who are going to shine through even more so, even earlier, than they might have otherwise."
Bhargava believes that the program plays a vital role in helping even clever students make an early transition from skilled problem solvers to researchers. "Duluth helps expose people to mathematical research, which is very different than mathematics in coursework and problem solving," he says. "When you take coursework, you never have to solve problems where you are thinking about something in a new way."
Because of a family event in India in the summer of 1995, Bhargava had to arrive in Duluth a few weeks late. Before he left, he spoke to Gallian by phone and asked if he could have his problem early. Gallian agreed, and while in India, Bhargava worked on the problem whenever he had a spare moment. By the time he arrived in Duluth, he had solved it.
Gallian responded with another, harder problem concerning polynomial mappings from Z/nZ (integers modulo n) to itself. The problem was to classify the types of mappings that can be expressed by polynomials. This one took longer, but Bhargava solved it, too, giving a complete description of such maps in the form of a formula.
The formula involved factorials, and he found himself wondering about their significance. By the end of the summer, he had come up with a generalized factorial function, work that drew the attention of the mathematical research community. At the end of the summer, he went back to Harvard and continued working on the problem for his senior thesis. He received both the AMS-MAA-SIAM Frank and Brennie Morgan Prize for Undergraduate Research and the MAA Hasse prize for his work.
Wood's Duluth research built on Bhargava's. She related his factorial functions to the general structure of the rings under the p-adic metric and proved that certain types of bases of integer-valued polynomials cannot exist. This work, along with her thesis work at Duke, earned her a Morgan prize as well. Gallian helped her with the application.
The Duluth program "was a big deal, because it made me feel like this was something I could do," Wood says. "It was something real and interesting: It wasn't undergraduate research, even though I was an undergraduate at the time."
A Day in the Life
Though he no longer does traditional research, one might still wonder whether Gallian's time-consuming REU activities cause him to shirk his duty to the students at the University of Minnesota.
Not so, he says, noting that this summer, while the program is running, he is also teaching pre-calculus.
Gallian is as popular with average students as with superstars. In his fourth year at Duluth, he received the University of Minnesota-wide Horace Morse award for outstanding teaching, and in 2003 he was named Minnesota Professor of the Year by the Carnegie Foundation for the Advancement of Teaching. He often teaches non-standard courses, such as a freshman seminar on mathematics and sports and an honors seminar on science and society. "UMD is a perfect fit because they allow me to do all these non-traditional things," he says. "They support whatever I do."
Gallian's most unusual professional activity may be his course on the Beatles. A big fan, he names Paul McCartney and John Lennon as two of his "all-time heroes."
Each year, Gallian's Beatles course is oversubscribed. Only the first 30 students who request the course are admitted automatically, but he accepts another 20 or so who make a good case as to why they should be admitted. "I want people who are knowledgeable about the Beatles," he says.
Ideally, they'll also be fun to spend a semester with.
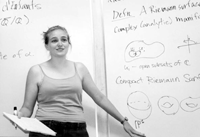
Melanie Wood, a participant in 2000, returned to Duluth for three summers as a visitor and this year as an adviser.
Sara Robinson is a freelance writer based in Pasadena, California.

