How to Look at Art
May 14, 1998Marc Frantz
The appreciation of art has so many aspects-including some that are probably ineffable--that a comprehensive discussion would be impossible. The title of this article may therefore seem presumptuous, and would probably be even more so to a person trained in the fine arts who was reading such a thing written by a mathematician. As someone whose undergraduate degree is a BFA with a major in painting, however, I can tell you that my former classmates and I could have benefited from a bit of mathematical advice along these lines.
In particular, if we had been a bit more canny about looking at our own works of art, we would in many cases have noticed drawbacks that, when corrected, would have resulted in more competent work. The advice I have in mind concerns the appreciation of artwork that employs perspective, a topic that obviously lends itself to a mathematical treatment. In this article I informally present one example from a set of simple procedures--easily remembered and requiring no tools or calculations--that can be used by viewers of works of perspective in galleries or museums.
Before setting up the problem, I would like to briefly describe the more general setting in which I began to think about such things. With the support of a National Science Foundation initiative called Mathematics and Its Applications Throughout the Curriculum (MATC), I am currently involved in the development of an interdisciplinary course called Mathematics and Art. (SIAM has lent its support to MATC.) My course co-developer, Annalisa Crannell, has taught Mathematics and Art for two years now at Franklin & Marshall College, and I began teaching it this fall at Indiana University Purdue University Indianapolis. Portions of the text I am writing have been used by Crannell at Franklin & Marshall, and also by Catherine Roberts in a course called The Science of Art at Northern Arizona University. Both instructors have provided me with valuable feedback and criticism.
Not surprisingly, I seem to score the most "hits" with students when I avoid grand mathematical generalizations, and instead focus on questions like "What kinds of things did you want to know in art school that were never clear to you?" It is mainly in this spirit that the material in the text is written, including tips on how to look at art.
Did I Really Draw a Cube?
A typical art student's experience with perspective drawing or, to be more honest, my own memory of this experience can be used to introduce the problem. Let's say I want to draw a cube. Remembering that something called "one-point perspective" can help, I decide to use it. I start by drawing the one point, called a vanishing point, and a square to serve as the front face of the cube (Figure 1(a)).
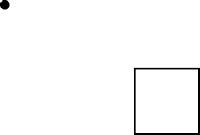
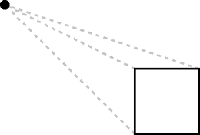
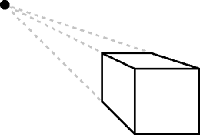
Figure 1. The author follows the first steps in the procedure for drawing a cube (a and b, left and middle), after which some guesswork leads to a dubious result (c, right).
Next, I lightly draw dashed lines from three corners of the square to the vanishing point (Figure 1(b)). At this stage things get a little vague. I need to draw a vertical line segment to represent the left rear edge of the cube, but where do I put it? Not being sure, I just plunge ahead and draw this edge by guessing, along with the other visible edges, and consider myself done, except for erasing the guide lines (Figure 1(c)). After looking at the other students' work, however, I find my cube a bit too elongated in the direction perpendicular to the front face. Did I really draw a cube, or something else?
The real problem, as you may have guessed, is that nowhere in my thought process did I consider the correct viewing position for an observer of the drawing. It somehow eluded me in art school that perspective is simply the result of projecting points from an object in three-dimensional space along straight lines (light rays) that strike one eye of an observer. The points where these lines pierce a "picture plane" constitute the perspective image of the object.
I think it would have helped quite a bit if we had performed a simple classic experiment like that shown in Figure 2, in which I am the observer, my student Pat Sullivan (at the window) is the projection function, and the window is the picture plane. By following my directions, Pat used masking tape to make a fairly decent perspective drawing of the IUPUI Library. A viewer who wishes to see the true shape of the library must place his or her eye exactly where mine was, or else the drawing will appear distorted; this is exactly the problem with the drawing of the cube.
It turns out that Figure 1(c) can be considered a correct drawing of a cube if (and only if) the viewing distance is specified to be three units, where one unit is the length of a front edge of the cube--a very small distance! Thus, the real problem with my drawing is that, in order to avoid seeing a distortion, the viewer is forced uncomfortably close to the page. If you can manage it, close one eye and place the other directly in front of the vanishing point in Figure 1(c), at a distance of about three units, and then glance off to the side at the drawing. If your eyes are no worse than mine, you should be able to make out a blurry but more cubelike shape than the elongated box that is perceived at a more natural--but incorrect--viewing distance. This problem is fairly common with beginning artists; their perspective drawings appear dramatic but "overperspectivized," because--unbeknownst to them--the correct viewing distance is unnaturally close to the paper.
I could have consulted any of the many texts on perspective, of course, and found just about anything I wanted to know about the correct way to draw cubes. Just knowing a little more about how to look at art, though, would have been sufficient to show me that my drawing needed improvement, and would have made me better able to appreciate the work of others.


Figure 2. The author (left) is the observer and the IUPUI Library the subject in a classic experiment on perspective drawing.
The Correct Viewing Distance in a Museum
Rather than show how to get the viewing distance for the cube, let's tackle a slightly more realistic example that uses a type of deduction that will be helpful when viewing art in a museum or gallery; you'll then be able to easily finish the cube problem yourself. Specifically, let us determine the correct viewing distance for the drawing of the house in Figure 3. (For the sake of brevity, some basic facts about one-point perspective drawing are presented here without proof. In the text for the Mathematics and Art course, these facts are proved by vector methods that can serve as a useful tool kit for students in other courses.)
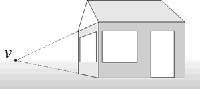
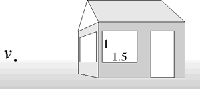
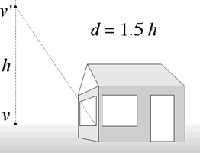
Figure 3. To determine the correct viewing distance for an observer of this drawing of a house (the front face of the actual house is assumed to be parallel to the picture plane), the author again follows a stepwise procedure; as with the cube in Figure 1, the viewing distance turns out to be impractically close.
Since the angles of the front face of the house appear undistorted (they are all right angles), we can assume that the actual house, which we imagine to be somewhere on the far side of the picture plane (the page), has its front face parallel to the picture plane. Thus, the lines determined by the top and bottom edges of the left wall are in reality orthogonal to the picture plane. The images of these lines in Figure 3(a) meet at the vanishing point v, and since the correct line of sight to the vanishing point must be parallel to the actual lines of the house, the correct viewpoint lies on a line through v, orthogonal to the page. The viewpoint can therefore be located once we know the viewing distance, and the viewing distance can be determined as follows:
-
Locate the vanishing point v in Figure 3(a).
- Find a rectangular feature on the left wall and determine its real-world width-to-height ratio r. We choose as our feature the window on the left wall, and make the assumption that it is in reality a copy of the window on the front wall. (Such an assumption is certainly valid for repeated architectural details like arches and carvings.) For the image of the front window, this ratio is estimated to be about 1.5 in Figure 3(b); the same ratio will apply to the actual front window and, by assumption, to the side window as well. Thus, we have r = 1.5.
- Locate the vanishing point v' of the diagonal of the feature chosen in step 2, and let h be the distance between v and v'. To do this, we use the following facts: Just as images of lines have vanishing points, images of planes have vanishing lines. The image of every line in a given plane has its vanishing point somewhere on the plane's vanishing line, so the plane appears to vanish at that line (e.g., the horizon line is effectively the vanishing line of a horizontal plane). The vanishing line for the plane of the left wall of the house is the vertical line through v, and since the diagonal of the window lies in this plane, v' is the intersection of the vanishing line and the image of the diagonal (Figure 3(c)).
- The correct viewing distance d is rh. In this case, the viewing distance is about 1.5h. This is true because (as mentioned earlier) from the correct viewpoint, a viewer's line of sight to the vanishing point of a line must be parallel to the actual line in space. By our estimate, the actual diagonal of the side window has an angle of elevation whose cotangent is r = 1.5; thus, the angle of elevation of the viewer's line of sight to v' must also have a cotangent of d/h = 1.5 (see Figure 4).
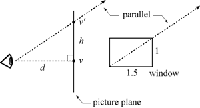
Figure 4. A viewer's line of sight to the vanishing point of a line must be parallel to the actual line in space (in this case the diagonal of the side window from Figure 3).
It's really as simple as a, b, c in Figure 3. Of course, you will not be able to draw lines on the works of art in a museum, but a good approximation to the correct viewpoint can be obtained with a little hand-waving and estimation (both time-honored activities). An almost equally simple method exists for determining the viewpoint of a two-point perspective drawing (the drawing of the library in Figure 2 uses two-point perspective), although space does not permit a discussion of it here.
In the house example, our analysis has again exposed a drawing whose viewing distance is so close as to be impractical, and this brings up an important point about original works in perspective that have been reproduced as figures in articles and textbooks: Such reproductions are not just miniaturizations-the potential difficulty for the viewer in assuming the correct viewing distance can actually make them distortions. In more than one way, there's no substitute for the real thing.
In Keeping with the Spirit of the MATC Initiative
I confess that I have had a great deal of fun deriving such techniques from scratch, because the process has dispelled much of the confusion that lingered from my art school days. These ideas are more than just fun, however. For instance, principles for looking at perspective art can be viewed as a subset of the important science of photogrammetry, which attempts to extract three-dimensional information from two-dimensional images.
By all accounts, students have also enjoyed the material on perspective, which includes things like theorems for drawing roofs and fences, and methods for making anamorphic art. I think such lessons are in keeping with the spirit of the MATC initiative, for one of the project's goals is to positively change the attitudes toward mathematics of students who are in traditionally nonmathematical disciplines. This particular goal is certainly achievable, for we have all had the experience of watching a seemingly dry piece of mathematics come to life when seen again in the context of a fresh and interesting application. In this respect, mathematics is much like art--it depends on how you look at it.
Marc Frantz ([email protected]) holds a BFA from the Herron School of Art in Indianapolis and an MS in mathematics from Purdue University. He is a lecturer in the Department of Mathematical Sciences at Indiana University Purdue University Indianapolis.

